
09. Circle of fifths
This figure is one of the most useful tools a composer can have. It gives a whole bunch of information, provided that we can read them. And that's what we'll see today
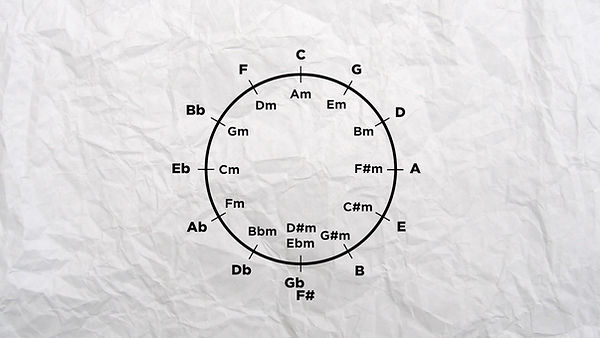
This figure is called the circle of fifths, because it show all the notes going from fifth to fifth. So the fifth of C is G, the fifth of G is D, the fifth of D is A, etc...
As all the notes are arranged by fifths, we can easily see what's the fifth of each note, by going clockwise on the circle.
And as a fourth is a fifth below the tonic, we can also quickly see the fourth of each note by going anti-clockwise on the circle. So the fourth of G is C, the fourth of C is F, the fourth of F is Bb, so on so fourth (pun intended).
But more that notes, this circle represent tonalities. And this circle allows you to quickly know which notes are in each tonality.
The tonality of C major, that is at the top, is made of only natural notes. Which means that it's all the white keys on the piano keyboard.
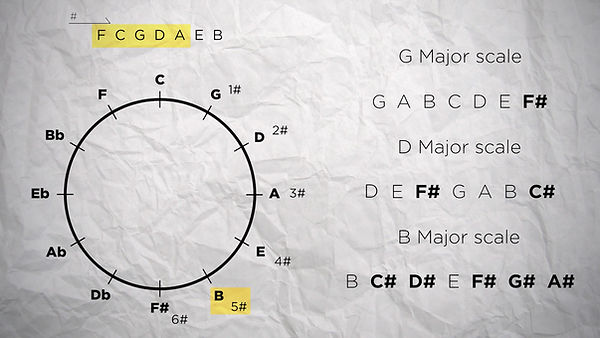
Then all the tonalities on the right side of the circle will have sharp notes in them.
Each step you go clockwise will add one sharp note. And the sharp notes appear in that order : F C G D A E B.
As a mnemonic to remember it, it's written there on the circle, from F to B

So the G major scale, begins with a G and will have 1 sharp note, which will be F#.
So the G major scale is G A B C D E F#.
The D major scale begins on D and will have 2 sharp notes, which are F# and C#. So that's D E F# G A B C#
One last example, the B major scale have 5 sharp notes, which are F# C# G# D# and A#. So the B major scale is B C# D# E F# G# A#.
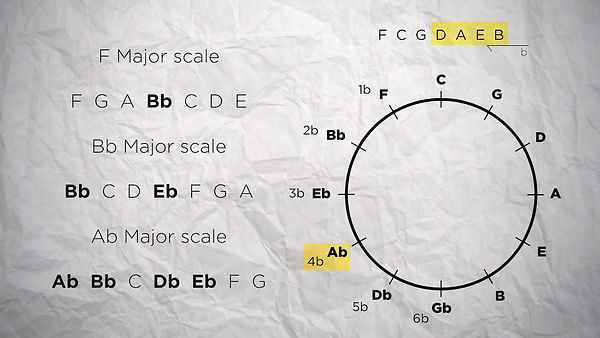
Then all the tonalities on the left hand side of the circle will have flat notes in them.
Same principle, each step you go anti-clockwise adds a flat note to the scale. And the flat notes appear in that order: B E A D G C F. Which is the exact opposite order of the appearance of the sharp notes.
So the scale of F major has one flat note, which is Bb. So the F major scale is F G A Bb C D E.
The scale of Bb major has 2 flat notes which are Bb and Eb, so the Bb major scale is Bb C D Eb F G A.
One last example: the scale of Ab major has 4 flat notes, which are Bb Eb Ab and Db, so the Ab major scale is Ab Bb C Db Eb F G.
Do you remember when I said "This figure can come very handy as a reference and it should definitely be on a cheat sheet if you need one"?
This is where it comes handy. Because knowing these chords of the C Major scale allows us to transpose them to the scales we just found on the circle of fifth.
We know the first chord would be Major, the second and the third degrees one would be minor, the fourth and the fifth degrees would be major, the sixth minor and the seventh diminished. So these are the chords you can use in the tonality of A flat major for intance.
In the same way you can find all the chords of all the major tonalities around the circle.

Now, looking at this circle of fifths, you should be able to find the notes of any major scale.
But it doesn't stop there. As we said in the video about scales, every major scale has a relative minor scale. This is a minor tonality that will use exactly the same notes than its relative major scale. The relative minor of C major is A minor for example. From there we can write all the minor tonalities on the circle, as they are all relative to a major scale.
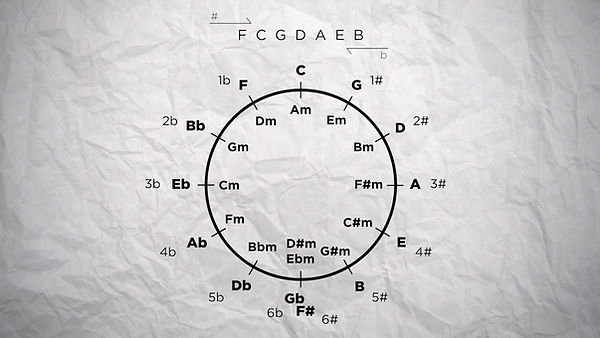
So in the same way that we did for the major tonalities, you can find the notes of all the minor scales.
For example the C minor scale has 3 flat notes, which are Bb Eb and Ab, so the C minor scale is C D Eb F G Ab Bb
And the C# minor scale has 4 Shap note, which are F# C# G# and D#, so the C# minor scale is C# D# E F# G# A B.
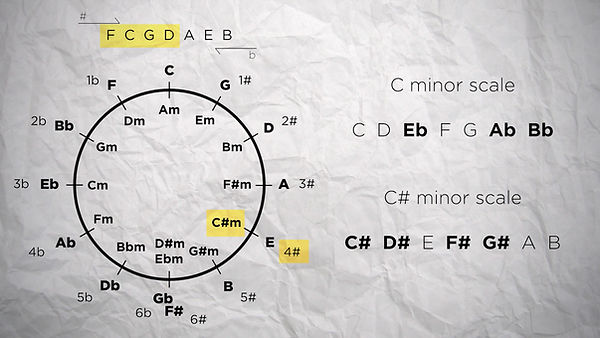
And in the same way we did with the major tonalities, you can know each chords of each tonality. We know the A minor scale uses the same notes than the C major scale, so it also uses the same chords, except the scale starts on the A. So these are the chords of the A minor scale, and we can transpose them to any tonality.
The Ist degree is minor, the 2nd is diminished, the 3rd degree is Major, the 4th and the 5th are minor, and the 6th and 7th degrees are Major.
This, for instance, are the chords you can use in a C# minor tonality.

So now we have all tonalities around the circle and we can quickly find the notes for each of them.
And it also works the other way around. You can find the tonality you are in from the notes you have in a melody. If you have a melody where all the Fs are sharp and all the other notes are natural, you're probably in G major or E minor.
If all the Bs and all the Es are flat, you're probably in Bb major or G minor.
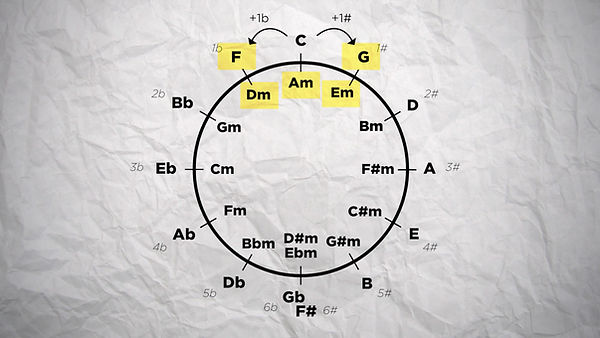
The way this circle is arranged, it groups similar tonalities together.
C major is close to G major because only one note, or rather one alteration separate them.
This comes very handy to make modulations, to switch from a tonality to another. Because the more notes two tonalities have in common, the easier it is to modulate from one to the other.
So from C major for instance, it should be very easy to make a transition to A minor, as All their notes are commons.
It should also be quite easy to go to G major, E minor, F major or D minor, as they all have only one note, or one alteration, that differ from C major.
These tonalities that are close to C major are called its adjacent scales.
A modulation to an adjacent scale is always smoother.
And it would be more difficult to make a transition between C major and F# major which very far on the circle, as there are a lot of alterations that separate them.
So when you are looking to do a modulation, the circle of fifth can be very handy to know the adjacent scales of your tonality, to know which tonality will be easier and make a smoother transition.
So if you are in B minor, the adjacent scales would be D major, E minor, G major, F# minor and A major. And in the same way you could find any the adjacent scales for any tonality.
And now I realized I haven't talked clearly about how to make these modulations, so that is what we'll talk about in the next video.