
03. Temperaments, how instruments are tuned
A trained ear can hear some of the harmonic overtones contained within one note. And these overtones are actually what was used to tune clavichords and other instruments. Especially the 3rd harmonic of the harmonic series, the fifth, the easiest to hear, considered to be the most consonant interval of all.
We used to tune our instruments fifth by fifth,
To have the fifth of a note you can multiply its frequency by 3, and then divide it by 2 (or 4) to lower it by one or two octaves.
So it's multiplying this frequency by 3/2 or 3/4.
So you would tune the first notes, let's say C (264Hz). Then, from C, you would tune the G which is the fifth of C. You can do that by ear, you'll need good ears, but you do that by listening to the "beatings" in the sound.
So from this G you could find the D. Then the A, then the E, then B, F#, Db, Ab, Eb, Bb and F.
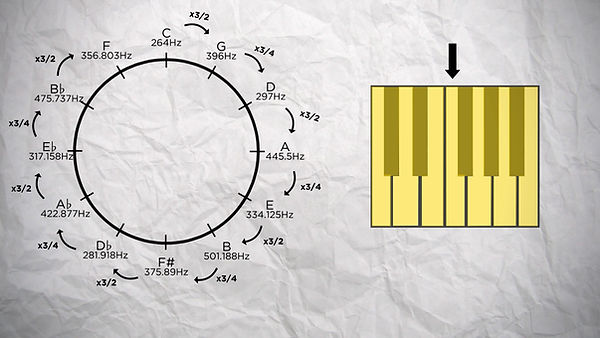
This figure is called the circle of fifths
The way we tune an instrument is called a temperament. This particular way of tuning an instrument, from fifth to fifth, is called the Pythagorean temperament.
It was made my Pythagoras himself. Yes, the same Pythagoras you heard of in middle school.
"He's considered to be the first one to theorise the harmonic series, and there, the first temperament."
(Note: this is not totally correct. Pythagoras worked on consonant intervals between two notes. For two strings of equal tension and thickness, he determined that they produced consonant notes when they are of the same length (unison), when one is half the size of the other (octave) or when one is 2/3 the length of the other (fifth). But he never talked about a "harmonic series".
This perfect fifth interval as described by Pythagoras was used to tune instruments, as described in this video. This way of tuning an instrument was called the Pythagorean temperament.)
That was the temperament used up to the XVth century, when occidental music used to favour fifth and forth, which was considered the only consonant intervals. But it came with 2 issues.

The first issue is that if we keep adding fifth, at the end of the circle, after the F we should land on a C. But the C we get by multiplying the frequency of our F isn't exactly the frequency we began with. This doesn't really aligns.
So this last interval has to be flatten so we can land on the C we're supposed to have.
In result, this interval becomes dissonant. It is called the wolf interval, because it reminds the scream of that animal.
The solution was to put this dissonant interval on an interval that was less used, or on an interval that we had to avoid carefully.
The second issue was that the thirds was not pure neither.
An interval of major third can be seen as 4 consecutive fifths.
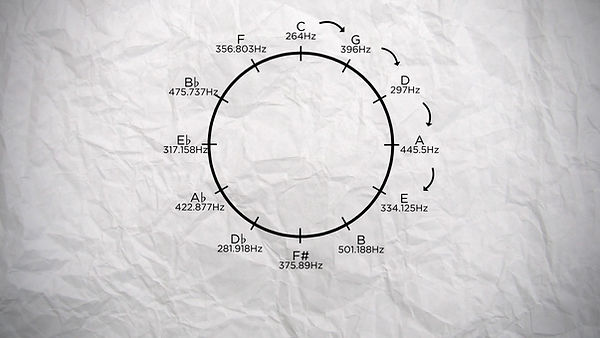
See there, from C to E for example. E is the major third of C, and there are four fifths between the two.
And if we compare the E in our circle here, which is the third of C, and the third that is in the harmonic series of C, the fifth harmonic overtone, we can see it that their frequencies are not equal neither.

It wasn't really a problem before the XVth century when the third was considered dissonant - and this tuning probably have something to do with that - but it became a problem when occidental music was tending to favour intervals of third and sixth.
The solution there was the meantone temperament. Or meantone temperaments I should say, because there are several.
The difference between the natural third found in the harmonic series and the third found in the Pythagorean temperament is called a comma.

And as the third is 4 consecutive fifth, the solution was to lower each fifth by 1/4 of that comma. So our thirds would be "pure", sacrificing the purity of the fifth in the process.
That was good enough for a music that favoured the thirds and sixths.
But the problem of the wolf interval was not resolved yet.
In fact this last fifth was below the "pure" fifth that we were supposed to have, and even farther from it. So the wolf interval was even worst.
But is also meant that the 4 thirds that was passing by this wolf interval were false as well, so you had to avoid all of them, and you had to avoid certain tonalities completely.
To minimize this problem, other meantone temperaments lowered each fifth by 1/6 or 1/8 of a comma, bringing the last fifth closer to a pure interval but sacrificing the purity of each third in the process. And any tuning was still bound to certain tonalities.
Because of that, for the next few centuries musicians has been creating different irregular temperament. Which made each tonalities sound differently due to the irregularities of the tunings.
That was until the XIXth century, when the equal temperament was created. That is the temperament we are still using today.
The principle of the equal temperament is that every note in the chromatic scale are separated by an equal interval.
This means that all the notes end up being slightly offset and all the intervals end up being slightly off as well.
Technically this tuning that we use today is the most inexact of all time.
But it makes all fifths and all thirds equally consonant as well.
So we don't have to avoid any tonality, they all get equivalent.
We lose the specific character that each tonality had in irregular temperaments but it allows us to travel from a tonality to another within the same music! Without having to avoid any of the tonalities neither.
As an egotist example, this is why my 98 chords song was even possible.
That also means that we can transpose any song to any key without having to retune our instrument.
So that's with this technically most inexact temperament of all that we get the more freedom. That what we gain from that equal temperament.
From there we can fiddle freely with our scales, and wander in any tonality,
And we'll surely see how to do that in other videos. In the meantime, thanks for watching and I'll see you next time.